Art of Islamic Patterns: Mughal Jaali

Arts & Culture
History

Reading time:4min

Written by Richard Henry 
Art courtesy of Art of Islamic Pattern

May 01, 2022

See the issue here: May-June-2022

Decorative and functional lattice screens have been produced by artisans throughout the Islamic world in a wide variety of styles. In India these are known locally as jaalis, a term that comes from an early Hindi word for “net.”

They are essential in the demarcation and articulation of architectural space. On a physical level, they moderate and modulate the passage of air and light, contributing to temperature, atmosphere and ambience. Composed using dynamic symmetries that are both unique and related to other traditions in the Islamic world, jaalis cast stunning patterns of evanescent light upon floors and walls of buildings.

India’s extreme climate, with its intense sun and heat, prompted the development of various styles of jaalis across the subcontinent through the centuries. They have been constructed in a range of media, including stone and plaster, in which inventive symmetries and distinct geometric devices proliferate.

For this third installment in the Art of Islamic Patterns series, we turn to Delhi, where the Isa Khan enclosure sits within the gardens of the funerary complex of Mughal Emperor Nasiruddin Humayun. It is named after Isa Khan Niazi, a nobleman in the courts of Humayun’s successors Sher Shah Shuri and Islam Shah Suri. The building was constructed in 1547-1548 CE with an octagonal layout characteristic of the style known as Lodhi. Alongside a balcony within its enclosure, an extraordinary, balustrade-height jaali of red sandstone displays a delicate, 12-fold radial design. Looking closely it shows beautiful interplay of 6- and 12-pointed stars as well as a subtle vibration that was created by overlapping figures that, although they might first appear to be circles, turn out to be 12-sided figures, or dodecagons.

As indicated in the first two articles in this series, geometric patterns based upon 3- and 6-fold symmetries are one of the three main families of such patterns with Islamic art, and thus they are one of the natural starting points for journeys into these patterns. Twelve is six doubled, and thus there is an essential interrelationship also between 6-fold and 12-fold designs. Both are typically derived from the same proportioning module, sometimes referred to as a “harmonic rectangle,” which is expressed in mathematical terms using a ratio of 1 to the square root of 3 (1:√3).

This harmonic rectangle has been used elsewhere, too: for example in the ground plans of the Merenid Bou Inaniya Madrasa in Fez, Morocco, and as a framing device within the illuminated pages of masterpiece Mamluk Qur’ans as well as, more generally throughout the Islamic world, a way to produce modular repeating units on geometric surface designs.

The photo below shows the module repeated four times, and the instructions that follow will guide you in the construction of one.

Richard Henry
Jaali screen from the Isa Khan enclosure of the funerary complex of Humayun, New Delhi. Red sandstone, 1547-1548.

What You Will Need

Compass: Choose one of high quality that will precisely hold a radius and for which you can keep a sharp point on the pencil lead.

Straightedge: A metal one works best, 30 to 50 centimeters in length.

Paper: Use smoothly finished drawing paper, at least A3 or 11 by 14 inches. For this pattern, you may wish to cut it into a square.

Eraser: Professional drafting erasers work best. Mistakes are part of learning to make patterns.

Pencils: Use hard leads, such as 2H, for lighter guidelines and soft leads, such as 2B and 3B, for heavier finishing lines. Add colors to fill as desired.

Tracing paper: A4 or 8½ by 11 inches size works well.

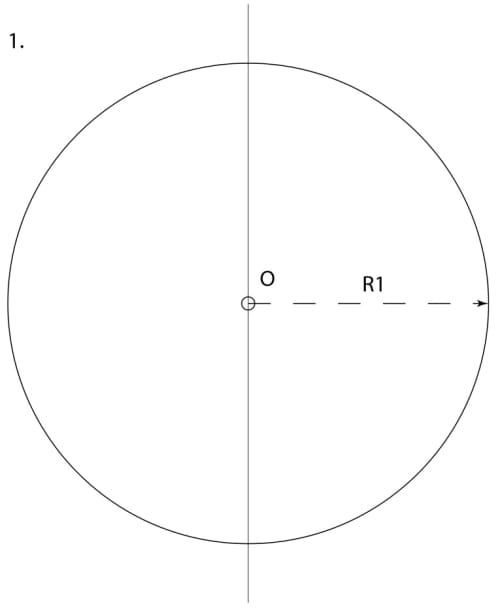
1. Start from a vertical line, set compass radius to R1, approximately ⅓ width of the page. Draw a circle in the middle of the line.

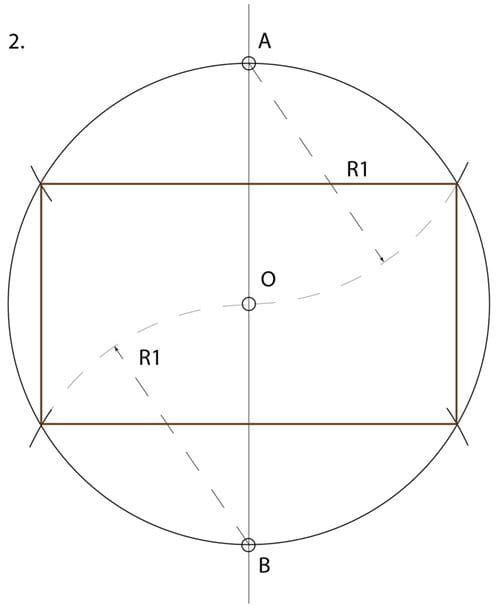
2. Without changing the compass radius, and with the compass needle anchored at point A then point B, make 4 additional divisions of the circle. This will create our “harmonic” proportioning rectangle with the special ratio of 1:√3. This will form the fundamental repeat module for our design.

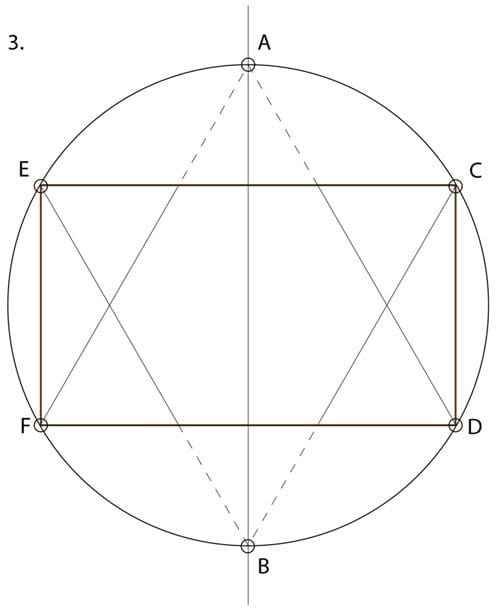
3. Link diagonal guidelines across our figure: A – F & D, B – E & C. Outside of the rectangle ECDF, these lines can be very light broken lines.

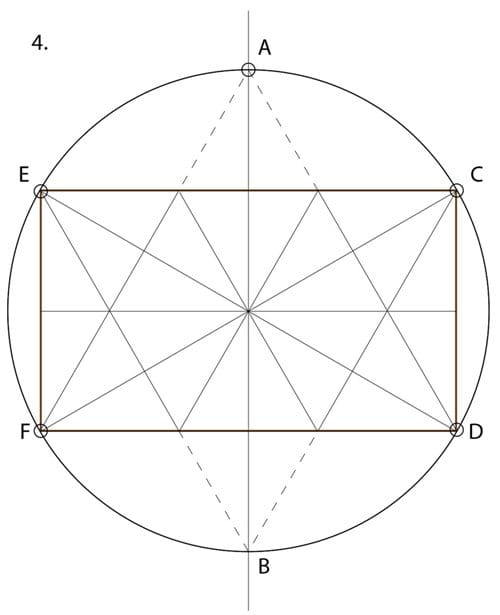
4. Add additional radial and diagonal lines, still as light guidelines.

5. Measure the distance of segment E-X. Then swing that arc to meet the line E-F at X1. Now measure a new compass radius R2 on the vertical line from F to X1. This will provide the radius of the key proportioning circles, which will contain the overlapping dodecagons in our final design.

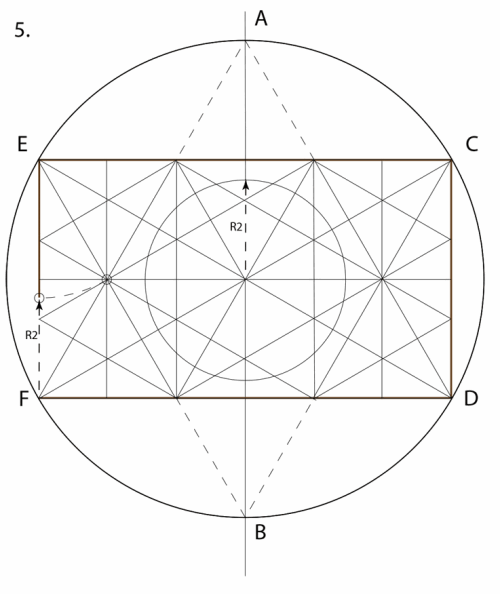
6. Arrange the proportioning circles with radius R2 evenly as above across our rectangular module. Note that two centers of the proportioning circle, G and H, are outside of the frame of our rectangular module.

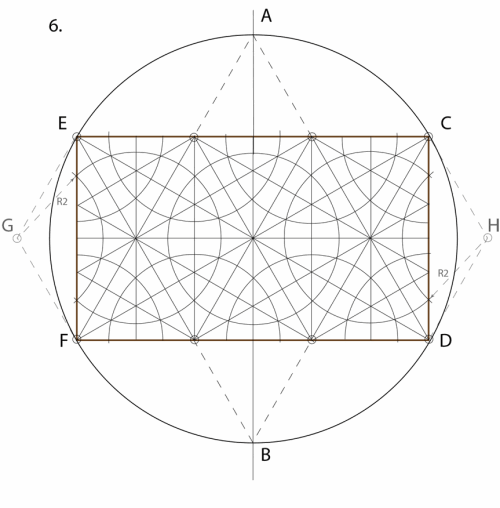
7. First lightly outline the 6- and 12-pointed star motifs in the center, as above. Then, with a bolder line, or colored pencil, start to outline the overlapping dodecagons and quarter dodecagons.

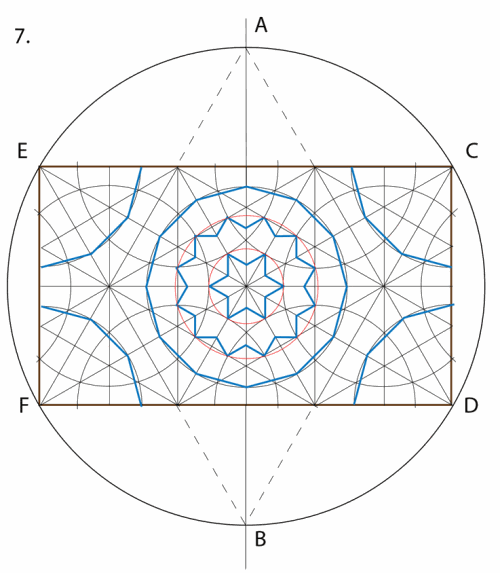
8. Note how additional proportioning circles centred at E, C, D and F enable us to establish the quarter stars nestled in the 4 corners of the rectangular repeat module. To complete the design, mark bold division with the larger gaps. The design can now be completed with shading, tone and colour to emphasise the interrelationship of different shapes and bring the design to life. Guidelines can be left as part of the final design or erased according to individual preference.